“Clearing Fractions” Clarified
Jerome Dancis remarks in “Toward Understanding and Remembering—How to do Hand Calculations with Fractions” that “It is not uncommon for students in college engineering calculus classes to still [be] doing calculations with fractions incorrectly. This reduces their chances for success.”, and offers several suggestions for dealing with this situation.
Most interestingly for my purposes right now, Dancis introduces what he calls “Epstein’s Rule”:
Epstein’s Rule. It is not all right to (or to teach students to) move numbers around in an equation.“Cross-multiplying” is cited as an example: students “learn” to solve“Subtracting 5 from both sides of an equation” uses the basic Rule: Equals minus equals are equals; instead of “moving the 5 to the other side” where it magically gets transformed to -5.
Violating Epstein’s Rule is an invitation and a common reason for creative mistakes.
This kind of mistake—or, more precisely, the persistence of such mistakes despite one’s efforts at clarification was the subject of two recent posts by me.
Which brings me to my purpose here today: to discuss a certain “with-understanding” method. One day it just sort of came to me.
They keep insisting on making the same mistakes over and over (quite often claiming “…but this is the way I was taught to do it”, as if a. at least one of their ex-teachers was a liar and b. that makes it alright to believe lies). I’m more-or-less duty bound to try to show them how to do it right. Nothing I’ve done so far ever seems to sink in. What haven’t I been doing? Well, the trouble seems mostly to arise in this “Clear Fractions” step … can I slow that down somehow and pin down more precisely where things are going wrong? Hmm … what if I use a Common Denominator and explicitly rewrite, e.g., as
before “Multiplying on Both Sides” (by 4, to obtain 2x = 3x -4 … which all my current students can then solve easily)?
In effect, the idea here is to combine two of Dancis’s “understanding” methods (multiplying on both sides of the equation and using common denominators). The amazing thing is, I’ve actually had a certain amount of success with breaking things down this way: I’m reasonably sure that, for a example, a small handful of my present students have made some progress in this area because I showed them this “extra step”. So I recommend it to whatever teachers may be struggling with this issue. Or would if I thought any were reading this.
-
1
Pingback on Feb 11th, 2008 at 5:26 am
[…] was wordier). One kid sets it up perfectly, just like we’ve been talking about over at Vlorbik’s: , and then everything went wrong. I stared for maybe 30 seconds before I understood (and I am […]
-
2
Pingback on Sep 22nd, 2020 at 4:11 pm
[…] Fractions and Student Incredulity trust no one02/07/08 “Clearing Fractions” Clarified move your pencil02/19/08 Radical Notations don’t […]
-
3
Pingback on Dec 14th, 2020 at 12:58 pm
[…] 02/01/08 Fractions and Student Incredulity trust no one 02/07/08 “Clearing Fractions” Clarified move your pencil 02/19/08 Radical Notations don’t […]
February 7, 2008 at 11:36 pm
You are so right! I have always hated the “cross-multiply” demon. My students often want to claim “cross multiplying” when then see fraction multiplication problems like 3/8 x 4/9. Never mind that there’s no equal sign in the middle — it looks almost exactly like the problems they call “cross multiplying.”
But it’s not just that. They get confused because they try to memorize a myriad of little specialized processes, when really the basic principles of equation solving and handling fractions will see you through every situation I can think of.
Basics, basics, basics. Thanks for the tip — I will give that a try next time I get a chance.
February 8, 2008 at 4:24 pm
evidently there’s been a flurry of activity
around the blathosphere about fractions.
who knew? (not me …) anyhow, th’ unapologetic
led me here; i’ll be checking it out
after class … or maybe *way* after …
next week say …
February 9, 2008 at 9:23 pm
I find my young students often know how to “cross multiply.” I begin using common denominators, and only slowly allow them to revert… Most do, but many stick with common denominators…
What you’ve done is exactly what I encourage.
Epstein’s rule, on the other hand, I do not agree with. The adding and subtracting from both sides (pendant addition) leaves many kids really really confused about signs. Since I began insisting on transposition, the number of errors I find has gone down dramatically. I do not allow students to “subtract from both sides”
Jonathan
February 10, 2008 at 9:28 pm
Well, I’m a teacher, and I read this. And “common denominator throughout then kill it by multiplying” is EXACTLY how I teach those sorts of fractions problems to 12-year-olds, as of the last two years. It seems to work pretty well, because, as you suggest, it falls back on a well-understood skill.
February 11, 2008 at 6:36 am
Jonathan,
What do you mean by “insisting on transposition” and “I don’t allow students to subtract from both sides”?
February 11, 2008 at 3:41 pm
the jd-ism “pendant subtraction”
seems to have been introduced in
this comment thread about a year ago.
i promote going from
7x + 3 = 17
directly to
7x = 14
without showing the subtraction explicitly
(but saying “subtract three from both sides”)
if somebody clearly isn’t getting it,
i’ll write down the two “-3″‘s under the equation
even though this bothers me slightly
(evidently not as much as it does jonathan).
i’d only ever write out
7x + 3 – 3 = 17 -3
explicitly if i were making some fancy point
(probably involving “additive inverses”),
February 11, 2008 at 10:28 pm
e,
I ban ‘hanging’ subtraction which seems to me to foul up kids work:
3x + 5 = x – 6
– 5 = -5
3x = x – 11
-x = -x
2x = -11
vs
3x + 5 = x – 6
3x – x = -5 -6
2x = -11
in my classes, latter only.
Jonathan
February 11, 2008 at 10:47 pm
Jonathan: my (weaker) classes are drilled to lay that out as follows:
3x+5 = x-6
[-5] 3x=x-11
[-x] 2x= -11
Though actually they would always “deal with” the x first.
February 12, 2008 at 2:09 pm
but what’s *really* annoying
is the way “pendant” calculation
messes up inequalities. for example:
-3x > 15 becomes
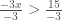 —
—
and these are of course *not* equivalent —
before mystically transmogrifying
(if we’re lucky) into (the correct)
x < -5.
and i don’t mind it that this *happens*.
i mind it that when i point out that it’s wrong
i’m, pretty much every time, considered
to be going out of my way to create
imaginary difficulties and i sacrifice some of
whatever little credibility i may have left.
February 13, 2008 at 2:44 pm
Vlorbik: yeah. I’ve very rarely seen calculations laid out in that way in the UK, but that certainly wouldn’t be an imaginary difficulty.
I’m not entirely decided on what my approach to multiplying through inequalities ought to be. With weak groups, I currently tell them they must only use positives and show them why. With stronger groups, I tell them that if they really want to they *can* use a negative and flip the sign (and of course explain how and why it works), but warn them it’s a favourite-mistake area. This policy is definitely negotiable from year to year…